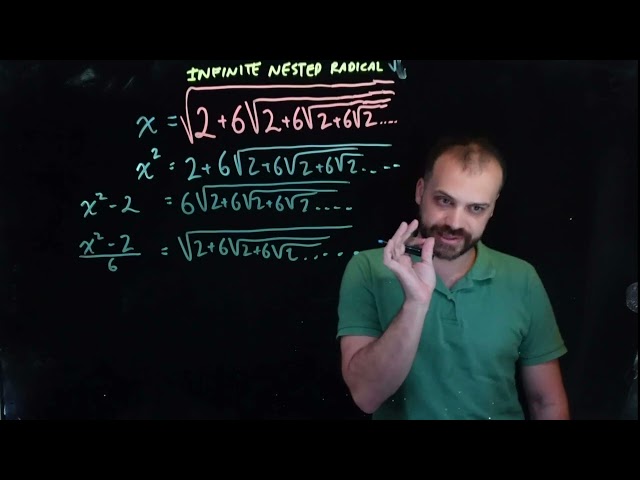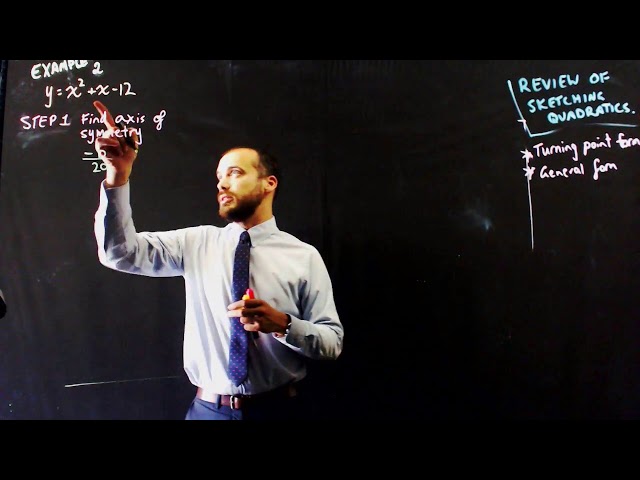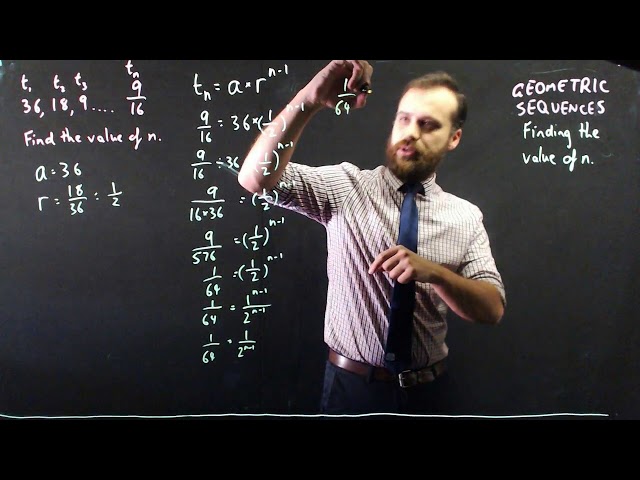Unit 1
Mathematical Methods (Queensland)
Topic 0: Surds
Note: This topic is NOT part of the current Mathematical Methods syllabus. However, the manipulation of surds is widely considered a useful skill (both in methods and specialist). For this reason, I include a short course on surds here.
-

Surds: What they are and why we need them
-

Proof that the square root is equal to the power of 1/2
-

Simplifying surds
-

Adding and Subtracting surds by grouping like terms
-

Multiplying surds
-

Surds and the distributive law
-

Dividing Surds
-

Surds Rationalising denominators
-

Rationalising denominators using conjugates
-

Infinite nested radicals
Topic 1: Arithmetic Sequences and Series
-

Sequence Notation
-

Recurrence Relations
-

Using a recurrence relation
-

Defining a sequence in terms of n
-

Applications of recurrence relations
-

Applications of recurrence relations non arithmetic
-

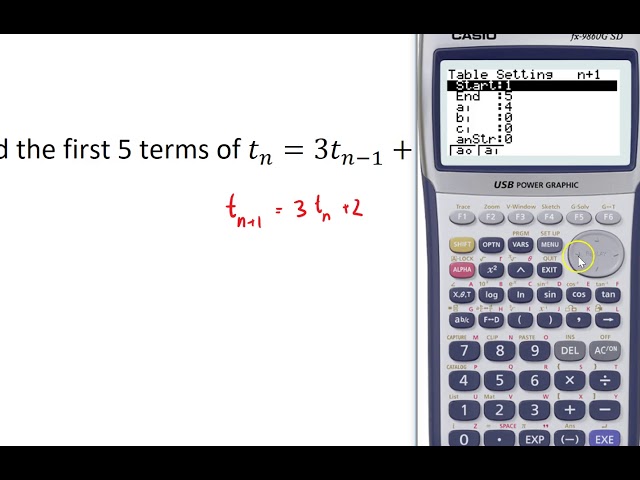
Using a calculator with recurrence relations
-

Arithmetic sequence formula
-

Rearranging the arithmetic sequence formula
-

Arithmetic sequences using simultaneous equations
-

Arithmetic sequences and series
-

Simultaneous equations using arithmetic series and sequences
-

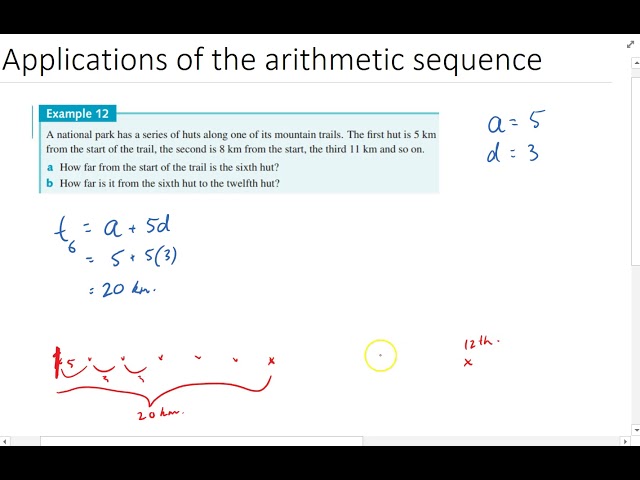
Application of the arithmetic sequence
-

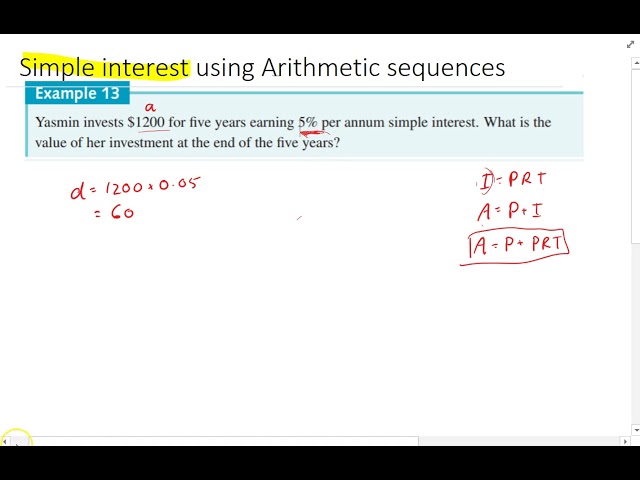
Simple Interest using arithmetic sequences
-

The Middle Term as the arithmetic mean in an arithmetic sequence
-

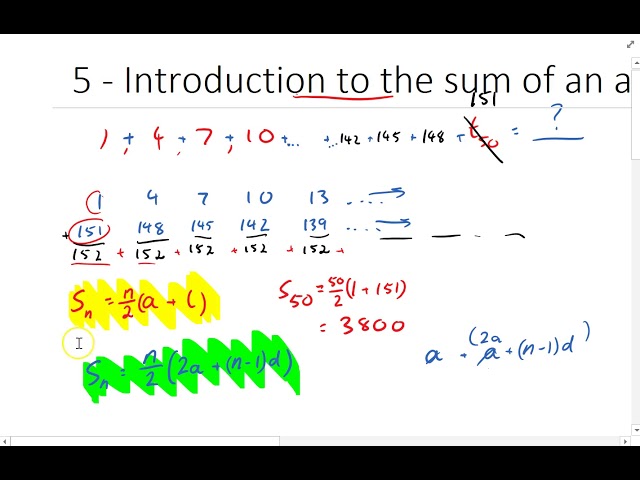
Introduction to the Sum of an arithmetic series
-

Arithmetic Series Formula Where it comes from and how to use it
-

Arithmetic Series resulting in a quadratic
-

Arithmetic Series and Simultaneous equations
-

Finding the number of terms in an arithmetic sequence
-

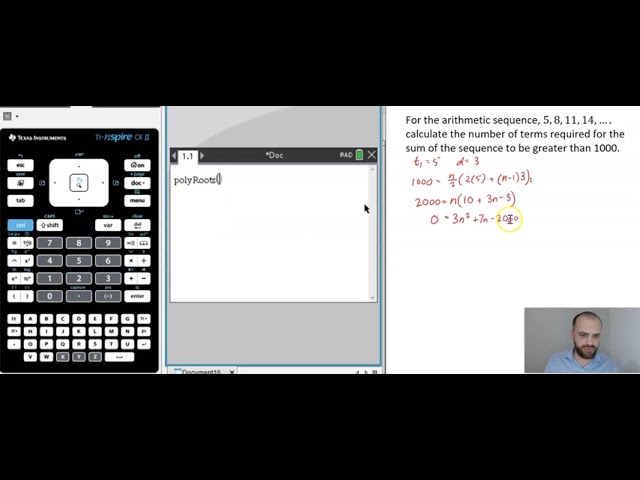
Arithmetic series Finding the number of terms that sum to a given number
-

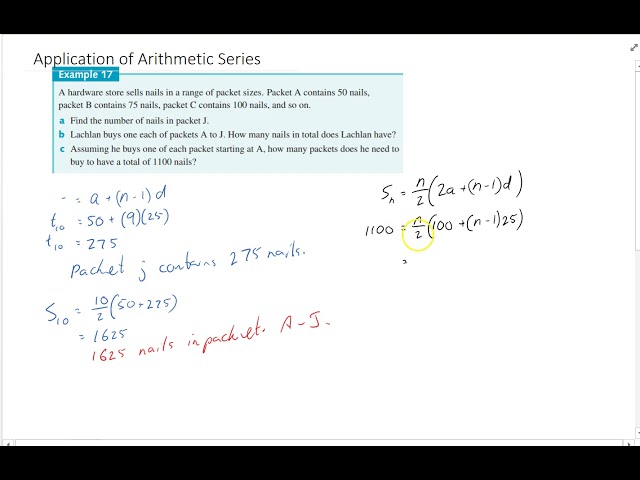
Application of arithmetic series
Topic 2: Functions and Graphs
-

Set Notation: Introduction
-

Interval Notation and Number Lines
-

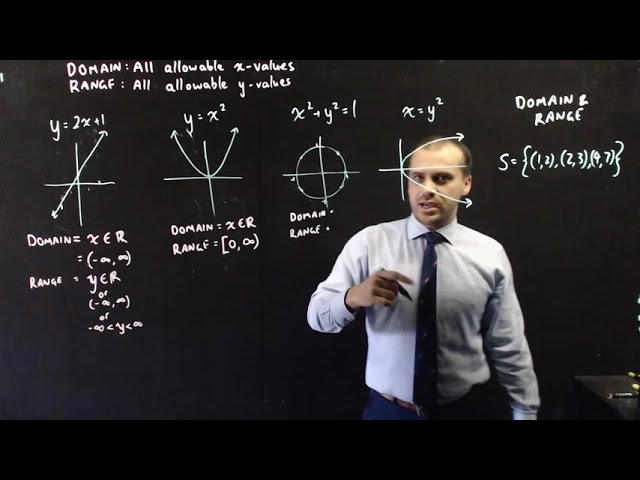
Relations Domain and Range
-

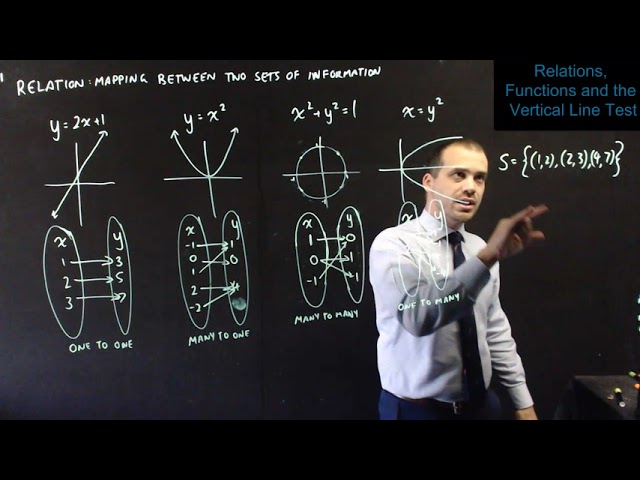
Relations, Functions and the vertical line test
-

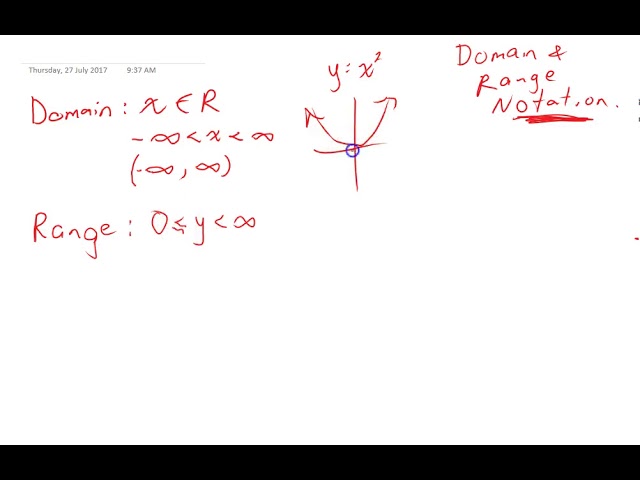
domain and range notation
-

Domain and Range of functions and relations
-

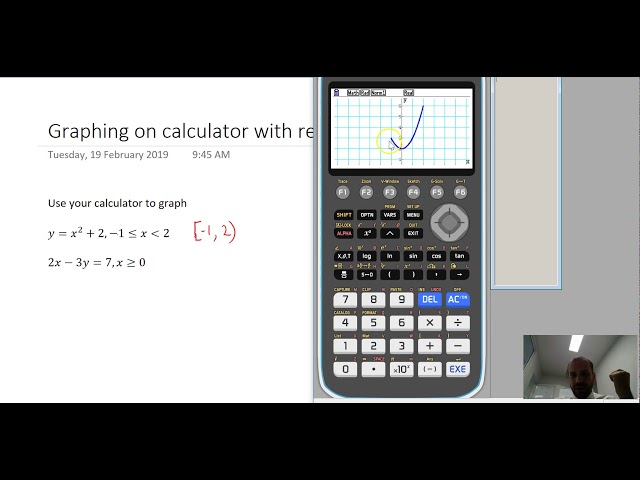
Graphing on calculator using restricted domains
-

What’s a function
-

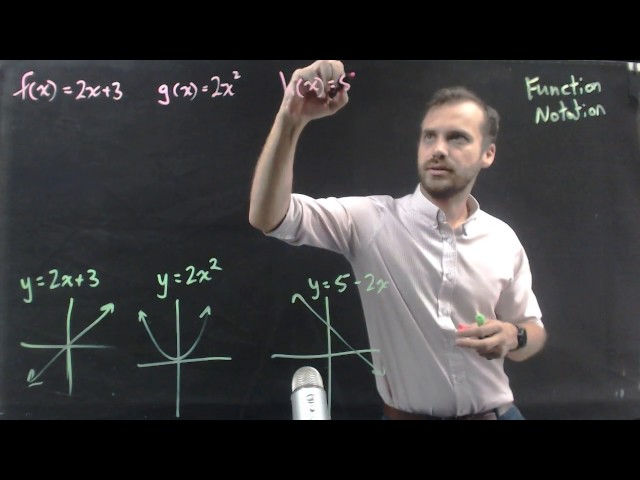
Function notation
-

Function Notation: Better ways of representing functions
-

Piecewise Functions
-

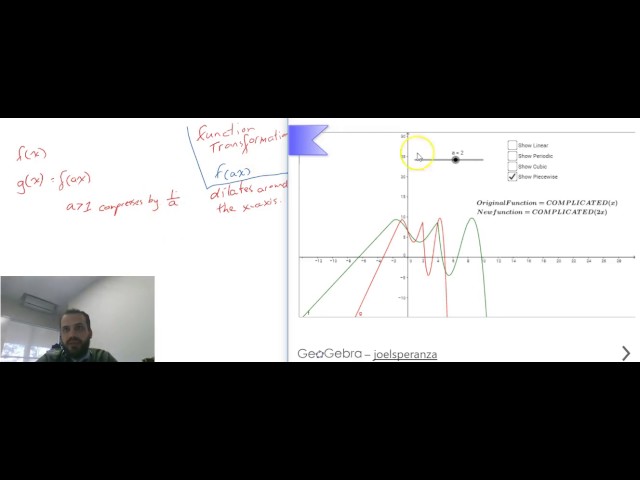
Function Transformations intro
-

Functions Transformation fx+a
-

Function transformation f(x+a)
-

Function transformation afx
-

Functions transformations f(ax)
-

Anatomy of a quadratic graph
-

Solving Quadratics 3 ways
-

3 forms of the quadratic equation
-

Review of sketching quadratics two ways
-

The quadratic formula and the discriminant
-

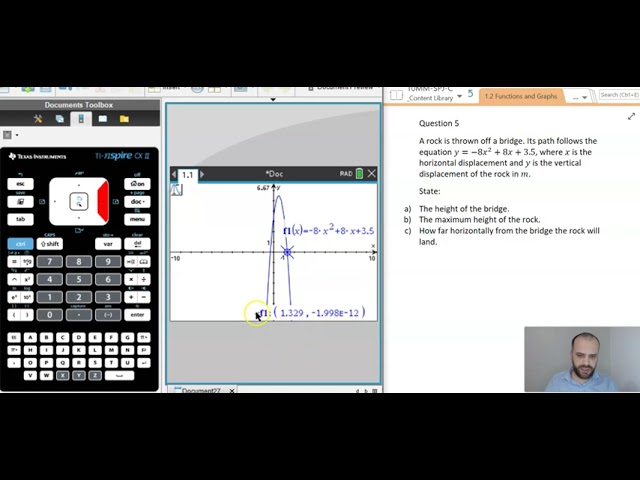
Modelling and problem solving with quadratics
-

solving simultaneous equations using quadratic and linear graphs
-

Direct vs inverse proportion
-

Equations of hyperbola and sketching
-

Finding equation of reciprocal function from sketch
-

Sketching Hyperbolas and why there’s an asymptote
-

Sketching circles and finding equations of circles
-

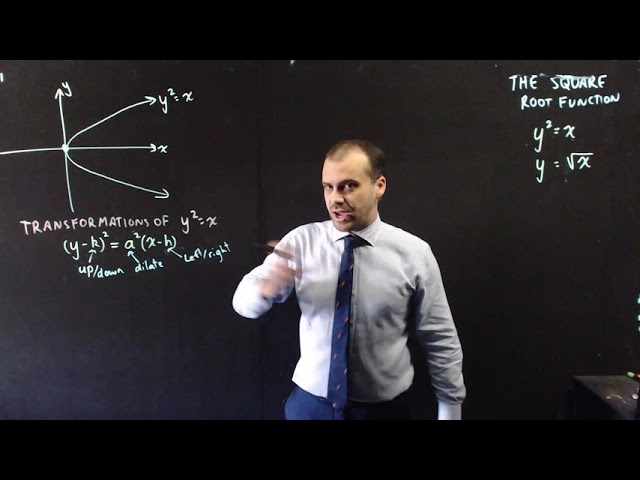
The square root function
-

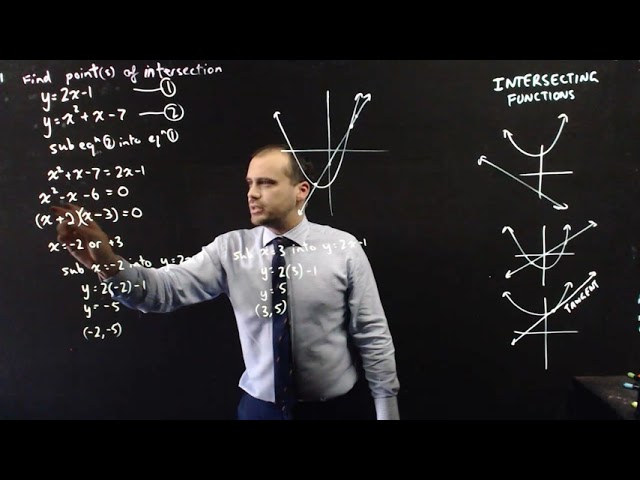
Intersecting Functions
-

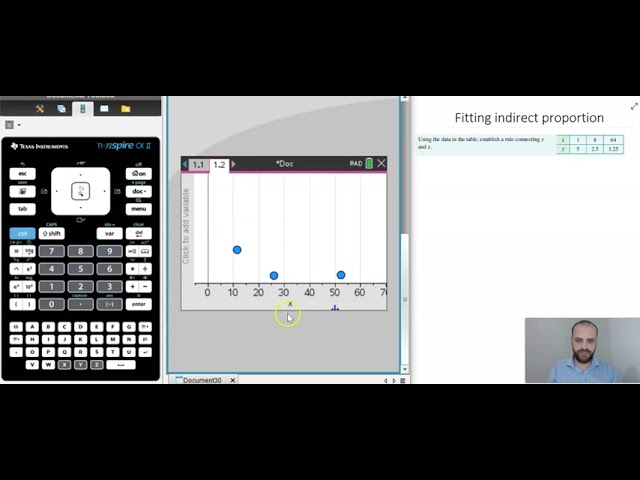
Direct and Indirect Proportion
-

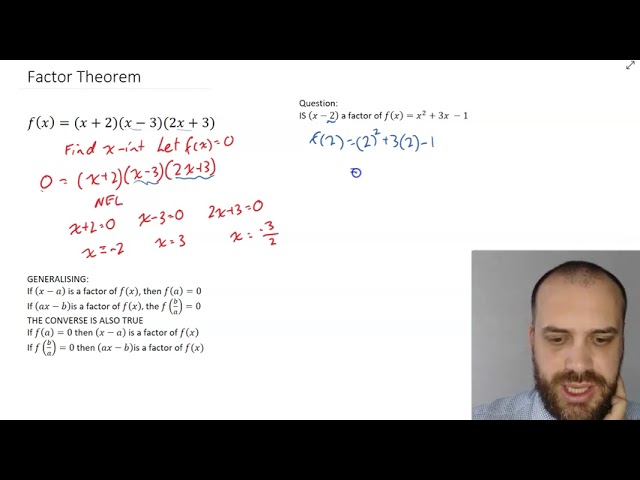
Factor Theorem Examples
-

Long Division: Learn this before polynomial division
-

Polynomial division
-

Finding a linear factor of a polynomial
-

Factorise solve and sketch a cubic
-

Cubics in “Turning Point” Form: (But it’s not a turning point)
-

Full quadratic review video
-

Fitting Indirect Proportion function to data using TI Nspire
-

Graphing and finding key points on the TI Nspire
-

Determining intersections of functions graphically on the TI Nspire
-

Sketching a graph on the TI Nspire
-

Solving simultaneous equations using LinSolve TI Nspire
-

Solving polynomials on the TI Nspire
Topic 3: Counting and Probability
-

The Language of Sets
-

Theoretical Probability with sets
-

All Probabilities sum to 1
-

Venn Diagrams the complement
-

Experimental probability
-

simplified tree diagram
-

The Addition rule of probability
-

Probability Tables intro
-

Conditional Probability formula
-

Conditional Probability Do you watch the bachelorette
-

Conditional probability rearranging the formula
-

Conditional Probability and Tree Diagrams
-

Independent events intro and tests
-

Independent events 2
-

Pascal’s Triangle and Selections
-

Binomial expansion using pascal’s triangle
Topic 4: Exponential Functions
-

Index Law 1 – multiplication of indices
-

Index Law 2 – dividing indices
-

Index Law 3 – raising to the power of zero
-

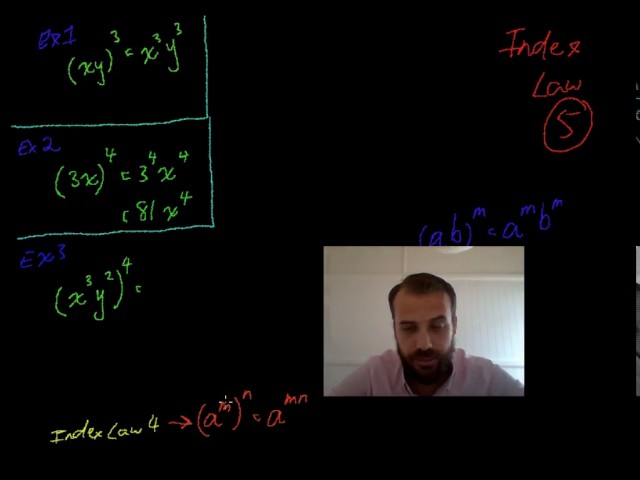
Index Law 4 – Raising a power to another power
-

Index Law 5 – worked examples using the fifth index law
-

Index Law 6 – expanding brackets around a fraction raised to a power
-

Index law 7 Negative Indices – converting Negative indices into positive indices using fractions
-

Index Laws Fractional indices
-

Index Laws Negative bases
-

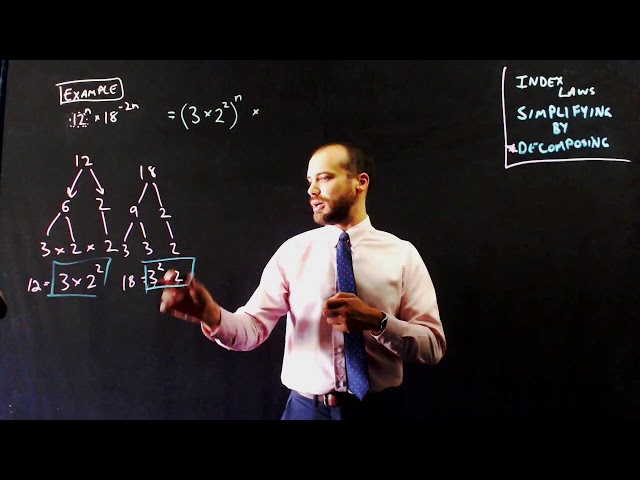
Index Laws simplifying by decomposing
-

Scientific notation Converting to scientific notation
-

Scientific Notation Convert scientific notation to decimal
-

Scientific notation Multiplying and dividing
Topic 5: Geometric Sequences and Series
-

Geometric sequences and the recursive definition
-

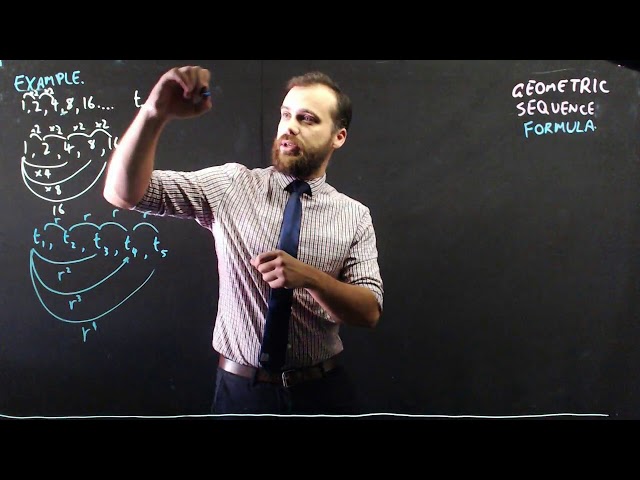
Geometric Sequence formula
-

Geometric sequences finding the common ratio
-

Geometric Sequences finding the value of n
-

Geometric Sequences and simultaneous equations
-

Geometric Sequences Geometric mean
-

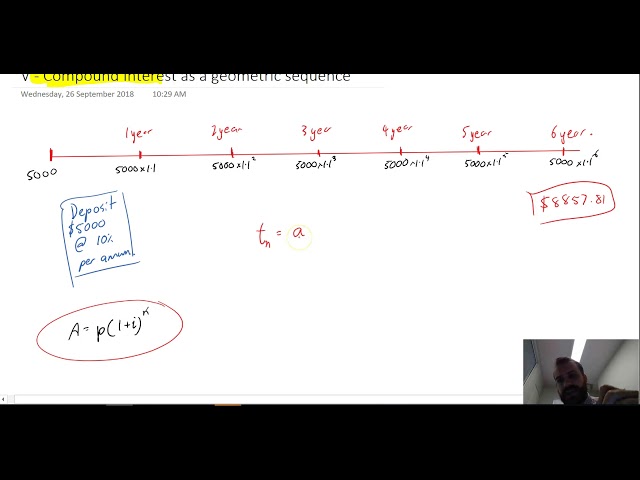
Compound interest as a geometric sequence
-

Geometric series formula proof and example
-

Geometric series find the value of n
-

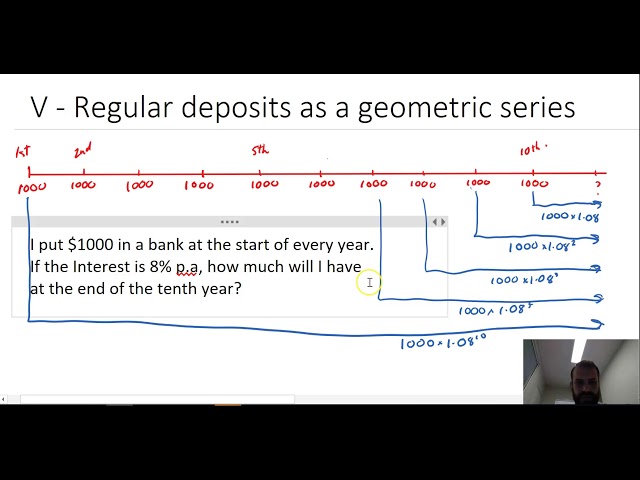
Regular deposits as a geometric series
-

Geometric Series Sum to Infinity and Zeno’s Paradox
-

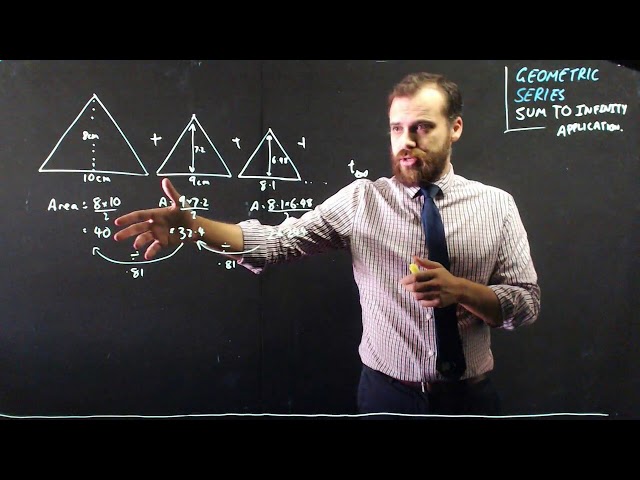
Geometric series sum to infinity application
-

Geometric Series Sum to infinity writing recurring decimals as fractions